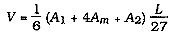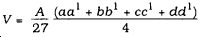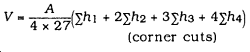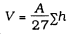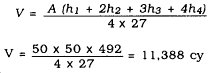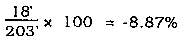CHAPTER 3
SURVEYS AND EARTHWORK OPERATIONS
Construction surveys are initiated when new construction is necessary. These surveys reveal the kinds of stakes to be used: provide data for earthwork estimation, including which method of estimation to use; and provide information for use on the mass diagram. The finished survey books should be filed with the construction project records of the Operations and Training Officer (US Army) (S3).
Earthwork operations are one of the most important construction aspects in road and airfield construction. Earthwork requires the greatest amount of engineering effort from the standpoint of personnel and equipment. Therefore, the planning, scheduling, and supervision of earthwork operations are important in obtaining an efficiently operated construction project.
CONSTRUCTION SURVEYS
Construction surveying is the orderly process of obtaining data for various phases of construction activity. It includes the following surveys: reconnaissance, preliminary, final location, and construction layout. The reconnaissance and preliminary surveys are used to determine the best location. The remaining surveys are conducted after a location has been established.
The purpose of construction surveys is to control construction activities. The number and extent of surveys conducted is governed by the time available, the standard of construction desired, and the availability of personnel and materials. In the combat zone, roads and airfields are constructed with only minimum preplanning and construction control activities. However, extensive surveys may be conducted for a deliberate project in the communications zone. The quality and efficiency of construction is directly proportional to the number and extent of surveys and other preplanning activities. The principles and techniques of field surveying are discussed in detail in technical manual (TM) 5-232 and FM 5-233.
After completing a thorough construction survey, transfer the design information from paper to the field by construction stakes. These stakes are the guides and reference markers for earthwork operations.
Mark the stakes so that the construction will conform to the planned line and grade of the road or airfield and the information on the stakes will be properly interpreted by construction crews.
RECONNAISSANCE SURVEY
The reconnaissance survey provides the basis for selecting acceptable sites and routes and furnishes information for use on subsequent surveys. If the location cannot be selected on the basis of this work, it must be determined by the preliminary survey.
PRELIMINARY SURVEY
The preliminary survey is a detailed study of a location tentatively selected on the basis of reconnaissance, survey information, and recommendations. It consists of running a traverse along a proposed route, recording topography, and plotting results. For roads, it may be necessary to conduct several preliminary surveys if the reconnaissance party has investigated more than one suitable route. Establish, station, and profile the route centerline with horizontal and vertical control points set. Take cross-section readings to allow rough calculations of the earthwork involved. (Sometimes cross sections may be taken during the reconnaissance survey if the conditions warrant.) If the best available route has not been chosen, select it at this time.
The airfield survey consists of establishing controls, noting terrain features, measuring glide-angle clearance, making soil profiles, and investigating drainage patterns and approaches. Accurately establish the final centerline during the survey.
FINAL LOCATION SURVEY
When time permits, conduct a final location survey. Establish permanent bench marks for vertical control and well-marked points for horizontal control. These points arc called hubs because of the short, square stake used. On most surveys, the hub is driven flush with the ground, and a tack in its top marks the exact point for angular and linear measurements. The hub location is indicated by a flat guard stake extended above the ground and driven at a slope so its top is over the hub. Hubs are 2 inches by 2 inches and the guards are flat stakes, about 3/4 inch by 3 inches.
Horizontal Control
The purpose of horizontal control is to accurately determine points for the various facilities of an engineering project. Establish permanent, well-marked points for horizontal control and reference them at the site before construction begins. On a large facility, establish a grid network and use it for this control. Tie the network into the military grid system in the particular area, if such a system has been established. On an airfield, place control points beyond the clear zone. These points define the centerline of the runway and other important sections of the airfield.
As the taxiways and other facilities are laid out, establish and reference new control points. In laying out the centerline, place target boards at each end of the runway so the instrument person can make frequent checks on alignment while the line is being staked out. Target boards may be set up on any line that requires precision alignment. Reference control stakes to ensure replacement, if they are disturbed or lost. Locate the target board just beyond the outermost control-point stake.
Vertical Control
Vertical control methods determine the difference in elevation between points. If available, establish a level reference surface or datum from a known bench mark. Differences in elevation, with corrections, are subtracted from or added to this assigned value, resulting in the elevation of the points. Take the datum of the bench mark system from a known elevation or barometer reading or make an arbitrary assumption.
CONSTRUCTION LAYOUT SURVEY
The construction layout survey is the final preconstruction operation. It provides alignments, grades, and locations that guide construction operations. The survey includes determining exact placement of the centerline; laying out curves; setting all remaining stakes, grades, and shoulders; staking out necessary structures; laying out culvert sites; and performing other work required to begin construction. Continue this survey until construction is completed.
CONSTRUCTION STAKES
Use construction stakes for centerline, slope, offset, shoulder, grade, reference, ditch, culvert, and intermediate slakes and for temporary bench marks. The stakes should be approximately 1 inch by 3 inches by 2 feet. Use finished lumber when possible. If it is not possible to use finished lumber, use small trees or branches blazed on both sides and cut to length. Finished grade stakes and temporary bench marks are 2 inches by 2 inches by 12 inches. Place stakes using a three-to five-person crew equipped with transit, level, rod, tape, ax, sledgehammer, and machete.
The primary functions of construction stakes are to indicate facility alignment control elevations, guide equipment operators, and eliminate unnecessary work. They also determine the width of clearing required by indicating the limits of the cut and fill at right angles to the centerline of a road.
Mark and place construction stakes to conform to the planned line and grade of the proposed facility. Use colored marking crayons to mark the stakes. Use a uniform system so the information on the stakes can be properly interpreted by the construction crew.
Construction stakes indicate--
- The stationing or location of any part of the facility in relation to its starting point. If the stake is located at a critical point such as a point of curvature (PC), point of intersection (PI), or point of tangency (PT) of a curve, note this on the stake.
- The height of cut or fill from the existing ground surface to the top of the sub-grade for centerline stakes or to the shoulder grade for shoulder or slope stakes.
- The horizontal distance from the centerline to the stake location.
- The side-slope ratio used on slope stakes.
The number and location of stakes used differ between roads and airfields. A typical set of construction stakes consists of a centerline stake and two slope stakes and is referred to as a three-point system. Point one is the centerline of the facility. Points two and three are the construction limits of the cut and fill at right angles to the centerline.
CENTERLINE OR ALIGNMENT STAKES
The centerline or alignment (hub) stakes, shown in Figure 3-1, are placed on the centerline of a road or airfield and indicate its alignment, location, and direction. They are the first stakes placed and must be located accurately. These stakes are used as reference points in locating the remaining stakes. Centerline stakes are placed at 100-foot (or 30-meter) intervals. On rough ground or sharp horizontal and vertical curves, place the stakes closer together. On horizontal curves, also stake the PC, PI, and PT. On vertical curves, also stake the point of vertical curvature (PVC), the point of vertical intersection (PVI), the point of vertical tangency (PVT), and the low point (LP) or high point (HP) of the curve.
Place centerline stakes with the broad sides perpendicular to the centerline. The side of the stake that faces the starting point is the front. Mark the front of the stake with a  for centerline and, if applicable, PC, PI, or PT. Also mark on the front the distance from zero or the starting point in 100-foot stations and the fractional part of a station, if used. For example, 6 + 5422 marked on a stake indicates it is 654.22 feet from the origin of the facility and is known as the station of this point. Stations are used in locating sections of construction and in preparing reports.
for centerline and, if applicable, PC, PI, or PT. Also mark on the front the distance from zero or the starting point in 100-foot stations and the fractional part of a station, if used. For example, 6 + 5422 marked on a stake indicates it is 654.22 feet from the origin of the facility and is known as the station of this point. Stations are used in locating sections of construction and in preparing reports.
Place the amount of cut or fill required at the station on the reverse side of the stake. A cut is marked C; a fill, F. A centerline stake, placed at station 78 + 00 and requiring a fill of 6.0 feet to bring this station up to the final grade line, would be placed and shown as indicated in Figure 3-1.
The amount of cut or fill indicates the difference between the final grade line and the ground line where the stake is emplaced. A point on the stake is seldom used as the line of reference to the final grade.
To prevent misinterpretation of the amount of cut or fill, mark decimal parts of a foot, as shown in Figure 3-1. The decimal part is written smaller, raised, and underlined. Facing the direction of increasing stations, the centerline forms the dividing line between the right and left sides of the area to be graded. When facing either side of the centerline, it is customary to refer to the areas as the right or left side.
SLOPE STAKES
Slope stakes, shown in Figure 3-2, define the limits of grading work. When used in read work, they can be used as guides in determining the width of clearing necessary. The area to be cleared usually extends 6 feet beyond the slope stakes. Set slope stakes on lines perpendicular to the centerline (one on each side), at points where the cut and fill slopes intersect the natural ground surface. Stakes at points of zero cut or fill are placed sloping outward from the centerline.
Sloping the stakes outward allows the equipment to work to the stake without removing it. The slope indicates the direction of the centerline of the road and enables the equipment operators to read the stakes more easily. Place slope stakes at 100-foot intervals on tangents and at 50-foot intervals on horizontal or vertical curves. Whenever a sharp break in the original ground profile occurs, it should be staked.
The front of a slope stake is the side facing the centerline. On this side of the stake, mark the difference in elevation between the natural ground elevation at this point and the finished grade at the edge of the shoulders. Under this figure, place another figure that indicates the horizontal distance from the centerline of the road to the slope stake. Place the station number on the other side of this stake. Below the station number, indicate the appropriate slope ratio. Figure 3-2 shows the proper markings for a slope stake in a typical situation.
OFFSET STAKES
Equipment used on a cut or fill section may destroy or remove many of the grade (centerline, shoulder, or slope) stakes. To prevent loss of man-hours and repetition of survey work, caution construction crews to protect grade stakes whenever possible. Place offset stakes beyond construction limits to avoid resurveying portions of the road to relocate these stakes. Figure 3-3 shows offset stakes used to relocate the original stakes.
Place offset stakes on a line at right angles to the centerline of the facility. From these, the slope stakes can easily be located. After relocating a slope stake, relocate the centerline stake by measuring toward the centerline of the road the horizontal distance indicated on the slope stake and placing the new centerline stake there.
An offset stake contains all the information given on the original slope stake plus the difference in elevation and horizontal distance from the original slope stake to the offset stake. Mark the offset distance on the front of the stake and circle it to indicate it is an offset reference. If the offset stake is at a different elevation from the slope stake, the cut or fill value must be increased or decreased by the difference in elevation. An offset stake placed a horizontal distance of 10 feet from and 1 foot above the right slope stake would be placed and marked as shown in Figure 3-3. Coordination between the surveyor and grade supervisor concerning the meaning of the markings is most important regardless of the type of marking used.
FINISH-GRADE STAKES
Use wooden stakes, 2 inches by 2 inches, with tops colored red or blue, for finish-grade stakes. Blue or red tops, as they are called, indicate the actual finished elevation of the final grade to which the completed facility is to be constructed. They are used when the grade is within a short distance of the final elevation. Do not use these stakes in combat road construction except in areas with steep slopes. This type of stake normally requires a guard stake to protect it and indicate its location. On large projects, it may be impractical to use guards with each stake.
There are no markings on finish-grade stakes other than the color on the top. These stakes may be set for use with the top of the stake exactly at the finished grade or with the top of the stake above the finished grade, as decided upon by the surveyor and construction foreman.
With the stakes set and marked at a predetermined distance above the finished grade, stretch a string between two stakes across the work and use a graduated ruler or stick to check the elevation. On an airfield layout, place these stakes along the centerline, edge of pavement, intermediate lines, shoulder lines, and ditch slopes. For road work, place stakes along the centerline and the edge of the shoulder; they may or may not be placed on the slopes.
REFERENCE STAKES
Many hubs marking the location of highways and airfields are uprooted or covered during construction. They must be replaced, often more than once, before construction is completed. AS an aid in relocating a point which may become hidden by vegetation, or as a means of replacing points which may have been destroyed, measurements are made to nearby permanent or semipermanent objects. This process is known as referencing or witnessing a point. On many surveys, permanent objects may not be available as witnesses. In such cases, additional stakes may be driven. These stakes usually are approximately 2 inches by 2 inches by 18 inches.
There are no markings on a reference stake. A point can be referenced by a known distance and a known angle or by two known distances. A transit must be used in the first case and may be used to advantage in the second. The method of using two known distances can be used, however, when a transit is not available. Place two points at measured distances from the point to be referenced. Use two tapes to relocate the original point or stake. Hold the zero end of one tape on one reference point and the zero end of the other tape on the other reference point. The point of intersection of the two tapes at the respective distances gives the location of the point in question.
To be of most value in replacing a missing station or point, the reference stakes or witnesses will be less than 100 feet from the point and, if possible, the arcs should inter sect at approximately right angles. Place them outside the construction limits, and indicate their location by blazing trees or additional stakes. Normally, the location of the reference stakes can be obtained from the surveyor's notebook.
CULVERT STAKES
Culvert stakes are located on a line parallel to and offset a few feet from the centerline. The information required on the culvert stakes includes the distance from the stake to the centerline, the vertical distance to the invert, and the station number. Once the survey crew has finished staking out the culvert, the construction supervisor can place the pipe accurately by using batter boards.
BENCH MARKS
Vertical control of a road or airfield must be maintained during construction. To do this, points of known elevation must be established. Obtain elevations from permanent monuments, known as bench marks, established by geodetic surveys. From these bench marks, run a line of levels and set temporary bench marks (TBMs). On small projects the TBMs frequently are set by running the levels from a point of assumed elevation. This is especially true of construction in combat areas.
Usually, TBMs are placed at 500- to 1,000-foot (or 150- to 300-meter) intervals and are placed off the limits of construction. Stakes 2 inches by 2 inches, solidly emplaced in the ground, may be used for this purpose. However, a nail driven into a tree, a manhole cover, or a pipe driven into the ground may also be used. Frequently, reference points serve as TBMs. The TBMs are set before setting the centerline stakes because vertical control must be established before construction begins.
EARTHWORK ESTIMATION
Earthwork computations involve the calculation of earthwork volumes, the determination of final grades, the balancing of cuts and fills, and the planning of the most economical haul of material. The exactness with which earthwork computations are made depends upon the extent and accuracy of field measurements, which in turn arc controlled by the time available and the type of construction involved. To plan a schedule, the quantity of earthwork and the soil and haul conditions must be known so the most efficient type and quantity of earthmoving equipment can be chosen and the appropriate time allotted.
When time is critical, the earthwork quantities are estimated either very roughly or not at all. When time is not critical, higher construction standards are possible and earthwork quantities are estimated and con trolled by more precise methods.
FUNDAMENTAL VOLUME DETERMINATION
The volume of a rectangular object may be determined by multiplying the area of one end by the length of the object. This relationship can be applied to the determination of earthwork by considering road cross sections at the stations along the road as the end areas and the horizontal distance between cross sections as the lengths. The end areas of the cross sections must be computed before volumes can be calculated.
METHODS OF END-AREA DETERMINATION
When the centerline of the construction has been located, measurements are taken in the field from which the required quantities of cut or fill can be computed. A cross-sectional view of the land is plotted from these measurements. The cross sections are taken on vertical planes at right angles to the centerline. Where the ground surface is regular, cross sections are taken at every full station (100 feet). Where the ground is irregular, they must be taken at intermediate points as determined by the surveyor. A typical cross section is shown in Figure 3-4.
Plot ground elevations from the surveyor's notes. Make a sectional template of the subgrade that shows the finished subgrade and slopes plotted to the same scale as the cross sections. Superimpose the template on the cross section and adjust it to the correct centerline elevation. Trace the template and extend the side slopes to intersect the original ground. If the section involves both cut and fill, draw only the appropriate lines of each template. When the sections are completed, begin the end-area measurements, then determine the volume. Of the several satisfactory methods of measuring the end areas, only the trapezoidal, stripper, double-meridian (triangular), and planimeter methods will be described in this manual. The method chosen will depend upon the time available, the accuracy desired, the aids at hand, and the engineer's preference.
Trapezoidal Method
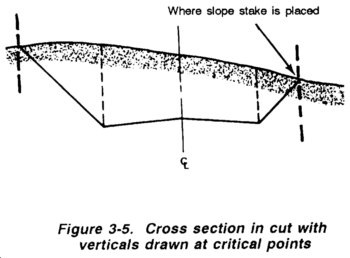
The trapezoidal method is widely used to determine end areas. The computations are tedious, but the results are accurate. In using the trapezoidal method, the area of any cross section is obtained by dividing the cross section into triangles and trapezoids, computing the area of each part separately, and taking the total area of the verticals to the ground line (Figure 3-5) in order to divide the cross section into two triangles and two trapezoids. Make the assumption that the ground is perfectly straight between these selected points on the ground line. While this is not usually correct, the assumption is within the accuracy normally required.
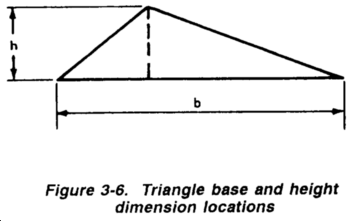
Basic Formulas. Before the area of the cross section can be computed, the basic formulas for the computation of the areas of triangles and trapezoids must be understood. If a line is drawn, as shown in Figure 3-6, from one of the vertices of a triangle perpendicular to the side or base (b) opposite this vertex, the line formed represents the altitude (h) of the triangle. The area of any triangle can be expressed as the product of one-half the base multiplied by the altitude. This relationship is expressed by the formula:
A = ½bh
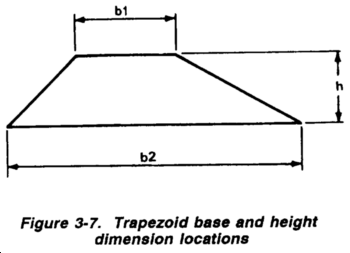
A trapezoid is a four-sided figure having two sides parallel but not equal in length, as shown in Figure 3-7. If the two parallel sides of the bases are crossed by a line perpendicular to each, the distance between the two bases along this perpendicular line is the altitude of the trapezoid. The area of any trapezoid can be expressed as the average length of the bases multiplied by the altitude. This relationship can be expressed by the formula:
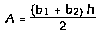
Computation of Areas. The first step in computing areas by the trapezoidal method is to break the cross-sectional area into triangles and trapezoids by drawing verticals, as shown in Figure 3-5. Then determine the area of these small figures by the appropriate formula.
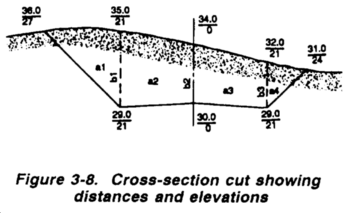
To determine the appropriate dimensions, the notes taken by the surveyors must be known. The cross-section notes taken in the field are in fractional form. The figure below the line indicates the horizontal distance from the centerline to that point on the ground. The figure above the line indicates the ground elevation of that point. Points on the grade line of the proposed road are written in a similar manner and are obtained by computations from the final grade line to be established, as shown in Figure 3-8. Thus, the note 32.0/21 indicates a point that is at elevation 32.0 and 21 feet from the centerline of the road. If the cross section is divided into triangles and trapezoids by erecting verticals, obtain notes for the centerline, shoulders, and end of slopes to solve for the area.
To solve the triangles and trapezoids formed, consider the bases of these figures to be vertical and the altitudes to be horizontal. All vertical bases are found by subtracting elevations, and all horizontal altitudes are found by subtracting horizontal distances from the closest vertical in the direction of the centerline.
Examples:
Referring to Figure 3-8, area a1, and substituting in the formula for the area of a triangle:
Referring to Figure 3-8, area a2, and substituting in the formula for the area of a trapezoid:
Find the areas of the remaining trapezoid and triangle in the same way.
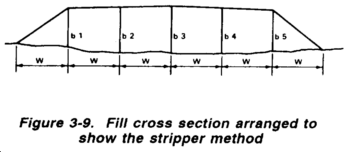
Stripper Method
The stripper method is a variation of the trapezoidal method. To use this method, consider a section such as that shown in Figure 3-9.
Example:
If vertical lines are drawn at equal distances apart, then by the trapezoidal formula, the end area, A, will be given by the following computation:
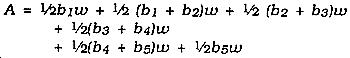
Factor in and combine terms:
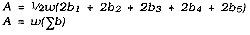
First, measure (graphically) each length (b) and multiply the sum by the width (w) (constant). The distance between vertical lines, w, may be any value, but it must be constant throughout the cross-section area. In rough terrain the vertical lines should be closer together to ensure greater accuracy.
One of the easiest and most convenient ways to measure the vertical lines (b) is with a strip of paper or plastic. Lay the strip along each vertical line in such a manner as to add each in turn to the total. The strip will show the sum of all vertical lines in the same scale that the cross section is plotted. This figure, multiplied by the value of w, will give the area of the cross section.
Inaccuracies result when either a triangle or trapezoid falls within the limits of w or when the area is curved. However, the method is rapid, and the accuracy is adequate under urgent conditions. Figure 3-10 shows a typical cross section with a stripper marked to show the total length of all vertical lines and the value of w. The stripper indicates that the sum of all vertical lines is 21.7 feet: w is given as 10 feet. Applying these figures to the formula, then--
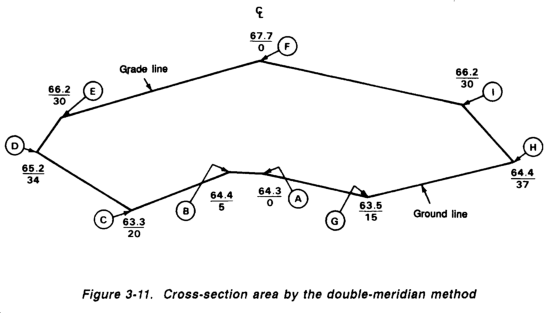
Double-Meridian Triangle Method
The double-meridian method explained in Chapter 13 of TM 5-232 gives a more precise value for a cross-section area than the stripper method. However, it involves more time.
With this method, shown in Figure 3-11, the area is subdivided into two series of trapezoids using the elevations of adjacent points and their projections on the centerline (the distances). These trapezoids have bases equal to the horizontal distance of the respective points from the centerline, and heights equal to their differences in elevation. Where the difference in elevation is plus, the area of the trapezoid is plus; where the difference is minus, the area of the trapezoid is minus. The component areas are added algebraically. Because this procedure uses the sum of the bases of the trapezoid, the area obtained is double the true area and must be divided by 2. The computation is simple arithmetic: subtract adjoining elevations, multiply by the distance from the centerline, add the multiplied results and list plus and minus quantities, add these quantities, and divide by 2.
The steps for completing the procedure for the double-meridian triangle method follow (refer to Figure 3-11).
1. Start at the centerline ground or grade elevation, whichever is lower (A). Work from the centerline in a clockwise direction to the left (A), (B), (C), (D), (E), (F); and counterclockwise to the right (A), (G), (H), (I), (F), to the centerline ground or grade elevation, whichever is higher (F).
2. Working from point to point, multiply the difference in elevation between each adjacent pair of points by the sum of their distance from the centerline. Point (F) to point (A) is not considered because the sum of their distances from the centerline is zero. Going from a lower to a higher elevation gives a plus quantity, while going from a higher to a lower elevation gives a minus quantity. Place plus quantities in one column and minus quantities in another.
3. Divide the algebraic sum of the plus and minus quantities by 2 to obtain the area of the cross section in square feet (sq ft). In sections having both cut and fill, treat each part as a separate section.
|
Example: The area of the cross section shown is computed as follows: Plus quantities: (A) to (B) (64.4 - 64.3) x ( 5 + 0) = 0.1 x 5 = 0.5 Total of plus quantities = 424.5 Minus quantities: (B) to (C) (63.3 - 64.4) x (20 + 5) = 1.1 x 25 = -27.5 Total of minus quantities = -39.5 Algebraic sum = 424.5 - 39.5 = 385.0 Area of section = 385.0 divided by 2 = 192.5 sq ft |
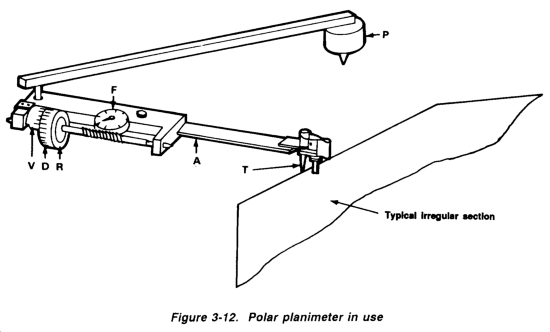
Planimeter Method
A polar planimeter is an instrument used to measure the area of a plotted figure by tracing its perimeter. The planimeter, shown in Figure 3-12, touches the paper at three points: the anchor point, P; the tracing point, T; and the roller, R. The adjustable arm, A, is graduated to permit adjustment to the scale of the plot. This adjustment provides a direct ratio between the area traced by the tracing point and the revolutions of the roller. As the tracing point is moved over the paper, the drum, D, and the disk, F, revolve. The disk records the revolutions of the roller in units of tenths; the drum, in hundredths; and the vernier, V, in thousandths.
NOTE: Always measure cut and fill areas separately.
Check the accuracy of the planimeter as a measuring device to avoid errors from temperature changes and other noncompensating factors. A simple method of testing its consistency is to trace an area of 1 square inch with the arm set for a 1:1 ratio. The disk, drum, and vernier combined should read 1.000 for this area.
Before measuring a specific area, determine the scale of the plot and set the adjustable arm of the planimeter according to the chart in the planimeter case. Check the setting by carefully tracing a known area, such as five large squares on the cross-section paper, and verifying the reading on the disk, drum, and vernier. If the reading is inconsistent with the known area, readjust the arm settings until a satisfactory reading is obtained.
To measure an area, set the anchor point of the adjusted planimeter at a convenient position outside the plotted area. Place the tracing point on a selected point on the perimeter of the cross section. Take an initial reading from the disk, drum, and vernier. Continue by tracing the perimeter clockwise, keeping the tracing point carefully on the lines being followed. When the tracing point closes on the initial point, take a reading again from the disk, drum, and vernier. The difference between the initial reading and the final reading gives a value proportional to the area being measured.
Make two independent measurements to ensure accurate results. The first is performed as discussed above. The second measurement is made with the anchor point again placed outside the area being measured but on the opposite side of the area from its position in the first measurement. This procedure gives two compensating readings the mean of which is more accurate than either.
To measure plotted areas larger than the capacity of the planimeter, divide the area into sections and measure each section separately, as outlined above.
Computer-Aided Design (CAD)
Very accurate measurements can be made if cross sections arc digitized using CAD. Cross sections can be placed on a digitizing pad, points plotted into the computer and, with one command, the area calculated.
METHODS OF VOLUME DETERMINATION
An engineer can accomplish the necessary earthwork computations by using the following methods: average-end-area, prismoidal formula, average-depth-of-cut-or-fill, grid, or contour.
Average-End-Area Method
The average-end-area method is most commonly used to determine the volume bounding two cross sections or end areas. Use the formula:
where--
V is the volume, in cubic yards (cy) (1 cy = 27 cubic feet (cf)), of the prismoid between cross sections having areas in square feet of A1 and A2, separated by a distance of L feet.
If cross sections are taken at full 100-foot stations, the volume in cubic yards between successive cross sections in square feet, may be found by the formula:
V = 1.85 (A1 + A2)
In either form, the formula is only accurate when A1 and A2 are approximately the same shape. The greater the difference in shape between the two end sections, the greater the possibility of error. However, the method is consistent with field methods in general. In most cases, the time required for a more accurate method is not justified.
Prismoidal-Formula Method
The prismoidal method is used where either the end areas differ widely in shape or a more exact method of computing volume is needed. Its use is very limited because it requires more time than the average-end-area method and gives greater accuracy than is required for most road and airfield construction.
The prismoidal formula is--
where--
V = volume (cy)
L = distance between end sections A1 and A2
Am = area of section midway between A1 and A2
Determine Am by averaging the corresponding linear dimensions of A1 and A2 then determining its area, rather than averaging the areas of A1 and A2.
Average-Depth-of-Cut-or-Fill Method
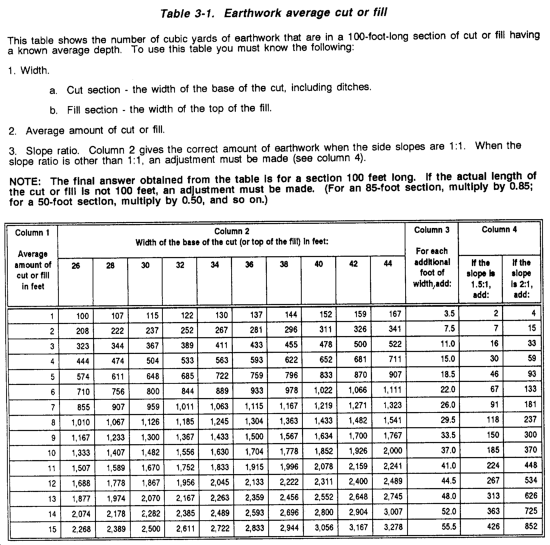
With only the centerline profile and final grade established, earthwork can be estimated with the average-depth-of-cut-or-fill method. Estimate the average depth of cut or fill between 100-foot stations and obtain the volume of material from Table 3-1. The accuracy of this method depends on the care given to establishing the centerline profile, the instruments used, and the accuracy of field reconnaissance.
However, the volumes obtained by this method are generally adequate for most military construction.
The centerline profile of a road is typical of the entire transverse section because of the narrow widths. Because of the greater width required on an airfield runway, the centerline profile may be misleading as to the typical conditions across the entire transverse width at that point. Therefore, earthwork quantities for airfields should be estimated mainly from cross sections. However, in the absence of sufficient time, the average-cut-or-fill method is better than none at all.
Determine the following before using Table 3-1:
- Average amount of cut or fill.
- Width of the base of the cut or the top of the fill in 2-foot increments between 26 feet and 44 feet.
- Quantity to be added to the figure in column 2, if the width of the base or top of the fill is an odd number of feet.
- Quantity to be added if the slope ratio on both sides is 1.5:1 or 2:1.
NOTE: The table is based upon a length of 100 feet between cross sections and a slope ratio of 1:1.
Follow these steps to use the table:
1. Enter column 1 and read down to the average amount of cut or fill for the length concerned.
2. Read horizontally to the right and obtain the figure under the appropriate base of the cut or top of the fill in column 2.
3. Make corrections to this figure from columns 3 and 4, if they apply.
4. If the length is not 100 feet between the points considered, adjust the answer proportionately.
Grid Method
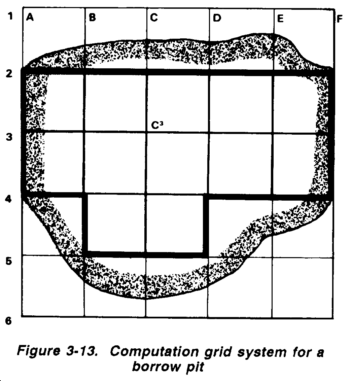
When the quantity of material within the limits of the cut sections is not enough to balance the fill sections, material must be borrowed. The most convenient method is to widen the cuts adjacent to the fills where the material is needed. Compute the volume by extending the cross sections. However, where this is not possible, locate borrow pits at some other area. The grid method is a convenient method of computing the borrow material available in a given borrow pit.
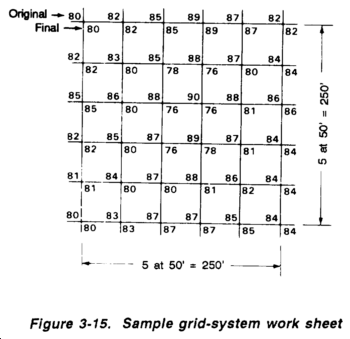
In this method, first stake out over the area a system of squares referenced to points outside the limits of work. The dimensions of these squares depend on the roughness of the original terrain, the anticipated roughness of the final surface, and the accuracy desired. Rougher terrain requires smaller dimensions to get accurate results. The squares must be of such size that no significant breaks, either in the original ground surface or in the pit floor, exist between the corners of the square or between the edges of the excavation and the nearest interior corner.
By taking elevation readings at the stakes before and after excavation, data is obtained to compute the volume of borrow taken from the pit. Figure 3-13 shows a borrow pit over which 25 squares were staked. To identify the various intersecting points, label lines in one direction by numbers and in 'the other direction by letters. Thus the intersection of lines C and 3 would be labeled C3.
Outline squares falling completely within the excavation with a heavy line. Within that line, determine the volume of excavation for each square in the following manner:
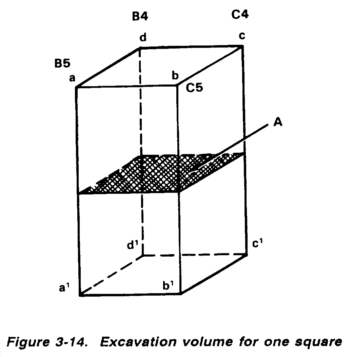
1. Label the points on one square, as shown in Figure 3-14.
2. Points a, b, c, and d are on the original ground line, while and are on the final ground line. The volume of the resulting form is the product of the right cross-sectional area A and the average of the four corner heights aa1, bb1, cc1, and dd1, in cubic yards.
3. The volume represented by each square might be computed by the preceding method and all volumes added. However, when a number of such volumes adjoin one another, it is quicker to use the following relation which gives the total volume, represented by all complete squares:
This could be approximated by adding all corner cuts and multiplying by A, or
In the preceding formula, A is the right cross-sectional area of one rectangular solid, h1 is a corner height found in one solid, h2 is a corner height common to two solids, h3 is a corner height common to three solids, and h4 and is a corner height common to four solids. As an example, is an h1, bb1 is an h2, dd1 is an h3 and cc1 is an h4. (Refer to Figure 3-13, and Figure 3-14.) The total borrow-pit quantity also includes the wedge-shaped volumes lying between the complete solids and the limits of excavation. For these volumes, use proportional surface areas. Use the formula:
A grid is illustrated in Figure 3-15. The length of the sides of each square is 50 feet. Therefore, given--
An alternative method is to compute the total of all cuts at each corner (123 feet), compute the average cut across all squares (123/25 = 4.92), and then multiply by the length of the sides of the figure.
FACTORS INFLUENCING EARTHWORK CALCULATIONS
On many projects, one objective of the paper location study is to design the grade line so the total cut within the limits of the work equals the total fill. The uncertain change of volume of the material make this difficult. It is usually more economical to haul excavated material to the embankment sections, thereby eliminating borrow and waste.
Shrinkage
Shrinkage has occurred when 1 cubic yard of earth, as measured in place before excavation, occupies less than 1 cubic yard of space when excavated, hauled to an embankment, and compacted. This difference is due to the combined effects of the loss of material during hauling and compaction to a greater-than-original density by the heavy equipment used in making the embankment.
Shrinkage is small in granular materials such as sand and gravel, and is large in ordinary earth containing appreciable percentages of silt, loam, or clay.
Shrinkage is very high (possibly 70 percent) for shallow cuts containing humus, which is discarded as unsuitable for embankments. These shallow cuts (usually 4 to 8 inches deep) are called stripping.
Loose and swell refer to a condition which is the reverse of shrinkage. The earth assumes a larger volume than its natural state when stockpiled or loaded into a truck. This factor ranges from 10 to 40 percent swell and is usually uniform for a given material.
Shrinkage, however, varies with changes in the soil constituents and with changes in moisture content and the type of equipment used. Consequently, a percentage allowance assumed in design may eventually prove to be 5 percent or more in error. A common shrinkage allowance is 10 to 30 percent for ordinary earth.
Settlement refers to subsidence of the completed embankment. It is due to slow additional compaction under traffic and to gradual plastic flow of the foundation material beneath the embankment.
Net Volume Calculation
Compute the volume of cut and fill and the net volume between any two points on the construction project. The net volume is the difference between the volume of cut and the volume of fill between any two specified stations. The net volume may apply to the entire project or to a few stations. Net volume may be described in a compacted, place, or loose state. Table 3-2 provides conversion factors used to find the net volume. All calculations are recorded on the earthwork volume sheet shown in Table 3-3.
Earthwork Volume Sheet
The earthwork volume sheets allow you to systematically record this information and make the neccessary calculations. They provide a means of tabulating earthwork quantities for use in the mass diagram discussed later in this chapter. The earthwork volume calculation sheet, shown in Table 3-3, is divided into columns for recording and calculating information.
Stations (column 1). List in column 1 all stations at which cross-sectional areas have been plotted. Normally, these areas are taken at all full stations and at intermediate stations that are required to fully represent the actual ground conditions and earthwork involved.
Area of Cut (column 2). Record in column 2 the computed cross-sectional areas of cut at each station. These areas may be computed by one of the commonly used methods, depending on the degree of accuracy required.
Area of Fill (column 3). Complete column 3 in the same manner as column 2, except show cross-sectional areas of fill.
Volume of Cut (column 4). Complete the volume of cut material between adjacent stations and record it in column 4. The most common method for computing volumes is the average-end-area method (or the earthwork table based on this method).
This volume represents only the volume of cut between the stations and the volumes reflected as in-place yardage.
Volume of Fill (column 5). Complete column 5 in the same manner as column 4, except show fill volumes. Fill volumes reflect compacted yardage rather than in-place yardage.
Stripping Volume in Cut (column 6). Before actual earthmoving operations begin, remove the layer of humus or objectionable material that lies on the ground surface.
This layer varies in depth but is usually 4 to 6 inches deep. This material must be wasted because it is not satisfactory to place in an embankment. Indicate in column 6 the volume between stations of this humus material over sections of cut.
Stripping Volume in Fill (column 7). Before an embankment can be constructed, the same layer of humus must be removed and the volume replaced with satisfactory material. Indicate in column 7 the volume of this material between stations over sections of fill.
Net Volume of Cut (column 8). Indicate in column 8 the volume of cut material between stations that is available for embankment. Column 8 is column 4 minus column 6, because the total cut must be decreased by the amount of material wasted in stripping, including the organic material.
Adjusted Volume of Cut (column 9). One cubic yard of material in its natural, undisturbed state occupies approximately 1.25 cubic yards when removed and placed in a truck or stockpile. The same 1 cubic yard, when placed in an embankment section and compacted, occupies a volume of approximately 0.9 cubic yards. In planning operations, convert these various volumes to the same state so the comparisons can be made, Changes in volume of earthwork are discussed in this chapter, and Table 3-1, provides the necessary conversion factors. Column 9 is column 8 multiplied by the appropriate conversion factor (in this case, 0.9) to convert it from in-place yardage to compacted yardage.
Total Volume of Fill (column 10). Indicate in column 10 the amount of compacted material required between stations to complete needed embankments. Column 10 is column 5 plus column 7, plus the amount necessary to replace the quantity removed by stripping. This figure represents the fill required to achieve the original ground line.
Algebraic Sum (column 11). Column 11 is the difference between column 10 and column 9. This indicates the volume of material that is available (plus) or required (negative) within the station increment after the intrastation balancing has been done.
Mass Ordinate (column 12). Column 12 indicates the total of column 11 starting at station 0 + 00. While passing through a stretch where cutting predominates, this column increases in value. While passing through a stretch where embankment is required, this column decreases.
THE MASS DIAGRAM
The first step in planning earthmoving plans operations is the estimation of earthwork quantities involved in a project. This can be done accurately by one of several methods, depending upon the standard of construction preferred. With these estimates, the engineer can prepare detailed plans for economical and efficient completion of the earthmoving mission.
The mass diagram is one method of analyzing earthmoving operations. This diagram can tell the engineer where to use certain types of equipment, the quantities of materials needed, the average haul distances and, when combined with a ground profile, the average slope for each operation. This permits the preparation of detailed management plans for the entire project. The mass diagram is not the complete answer to job planning, and it has limitations that restrict its effectiveness for certain types of projects. However, it is one of the most effective engineer tools and is easily and rapidly prepared.
CONSTRUCTION OF THE MASS DIAGRAM
Using column 1 (station) and column 12 (mass ordinate, cumulative total) of a completed earthwork volume sheet, a mass diagram can be plotted as shown in Figure 3-16.
Plot the mass diagram on scaled graph paper with the stations indicated horizontally and the mass indices (column 12) denoted vertically. Connect all plotted points to complete the mass diagram as shown in Figure 3-16. Positive numbers arc plotted above the zero datum line, negative numbers below.
PROPERTIES OF THE MASS DIAGRAM
Figure 3-17 shows a typical mass diagram with the actual ground profile and final grade line of the project plotted. Note that both use the same horizontal axis (stations). The ground profile is placed above the mass diagram to facilitate the calculation of the average grade over which equipment will work. The horizontal axis is the only thing these graphs have in common.
The mass diagram is a running total of the quantity of earth that is in surplus or deficient along the construction profile. If at one station more material is being cut than filled, you have a cut operation at that station. The quantity or volume of surplus material will be increasing as cutting operations continue through the station, producing an ascending mass diagram curve line. Cutting is occurring from stations A to B and stations D to E in Figure 3-17. The total volume for the cut at station A to B is obtained by projecting the points on the curve line at stations A and B to the vertical axis and reading the volume  .
.
Conversely, if at one station more material is being filled than cut, you have a fill operation at that station. The quantity or volume of deficient material will be increasing as filling operations continue through the station, producing a descending mass-diagram curve line. Filling is occurring from stations B to D in Figure 3-17. The total volume for the fill at stations B to D is obtained by projecting the points on the curve line at stations B and C to the vertical axis and reading and adding the volumes above and below the zero datum line.
The maximum or minimum point on the mass diagram, where the curve changes from rising to falling or vice versa, indicates a change from cut to fill or vice versa. This point is referred to as a transition point (TP). On the ground profile, the grade line crosses the ground line at the TP, as illustrated at stations B and D.
When the mass diagram crosses the datum line or zero volume, as at station C, there is exactly as much material filled as there is material cut, or zero volume excess or deficit at that point. The section of the mass diagram, from the start of the project at station A to a point of crossing the zero volume line, is known as a node. Each crossing point on the zero volume line indicates another node. The last node may or may not return to the zero datum line. Nodes are numbered from left to right.
The final position of the mass diagram line, above or below the datum line, indicates whether the project was predominately cut or fill. In Figure 3-17, where the mass diagram ends at station E, the operation was cutting; that is, surplus material was generated by cutting and must be hauled away (waste operation). Borrow operations occur when the final position of the mass diagram is below the zero volume line.
PROJECT ANALYSIS
Once the basic properties of the mass diagram arc understood, the engineer can conduct a detailed analysis 10 determine where dozers, scrapers, and clump trucks will operate This is accomplished by using balance lines. A balance line is a line of specific length drawn horizontally, intersecting the mass diagram in two places. The specific length of the balance line is the recommended working or maximum haul distance for different pieces of equipment. The term maximum haul distance is used because operating the equipment beyond this point would not be efficient. The maximum haul distances (balance-line lengths) are--
|
Equipment |
Maximum Haul Distance |
|
Dozer |
Up to 300 feet |
|
Scraper |
301 to 5,000 feet |
|
Dump Truck |
5,001 feet to several miles |
These lengths are measured using the horizontal scale (stations measured in hundreds of feet). Always use the maximum haul distance (length) of each piece of equipment, provided the project or node is at least that length. Start excavating each node with the dozer followed by the scraper and dump truck.
Figure 3-18 shows a balance line drawn on a portion of a mass diagram. If this was a dozer balance line, the distance between stations A and C would be 300 feet.
Cut equals fill between the ends of a balance line. The mass line returns to exactly the same level, indicating that the input and the expenditures of earth have been equal. In Figure 3-18, this occurs between stations A and C. There has been an exact balance of earthwork.
In Figure 3-18, the amount of material made available by cutting between stations A and B is measured by the vertical line marked Q. This is also the amount of embankment material required between stations B and C. This is described as the balanced quantity of earthwork.
If equipment was used to do the balanced earthwork between stations A and C, the maximum distance that earth would have to be moved would be the length of the balance line AC.
In accomplishing balanced earthwork operation between stations A and C, some of the haul distance would be short, while some would approach the maximum haul distance. The average haul distance (AHD) is the length of the horizontal line placed midway between the balance line and the top or bottom point (transition point) of the curve (Figure 3-18) and is found by dividing the vertical distance of Q in half.
If the curve is above a balance line, the direction of haul is from left to right. The converse is true when the curve is below a balance line.
Figure 3-19 shows a part of a mass diagram on which two balance lines have been drawn. The same principles apply for the area between the lines as with only one balance line. The quantity balanced is the vertical distance between the balance line, while the horizontal bisector is the average haul distance. The longer balance line is the maximum haul distance, and the shorter balance line is the minimum haul distance. The haul distance depends upon the position of the curve with respect to the balance lines.
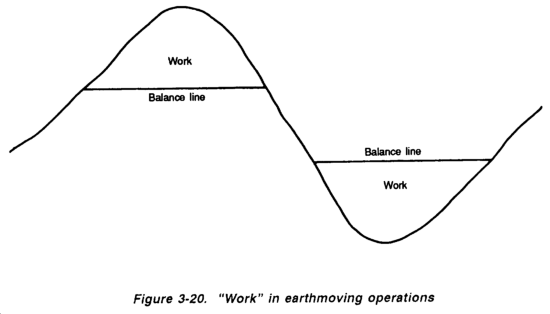
The mass diagram is a useful indicator of the amount of work expended on a project. By definition, work is the energy expended in moving a specified weight a given distance. It is the product of weight times distance. Because the ordinate of the mass diagram is in cubic yards (which represents weight) and the abscissa is in stations or distance, an area on the mass diagram represents work. In Figure 3-20, if equipment is used to do the balanced earthwork between the ends of the balance lines as drawn, the work expended is equal to the area between the mass line and the balance line.
Another item calculated from the mass diagram is average grade. This value is used when computing equipment scheduling and utilization. Figure 3-21 and Figure 3-22, illustrate a portion of the mass diagram on which the average grade has been determined.
USE OF THE MASS DIAGRAM
The mass diagram is used to find the cost of a project in terms of haul distance and yardage, to locate the areas for operation for various types of equipment, to establish the requirements for borrow pits and waste areas, and to provide an overall control of required earthmoving operations. However, the means used to analyze the mass diagram will follow the same principles regardless of the end result desired. The analysis of the mass diagram is based upon the proper location of balance lines.
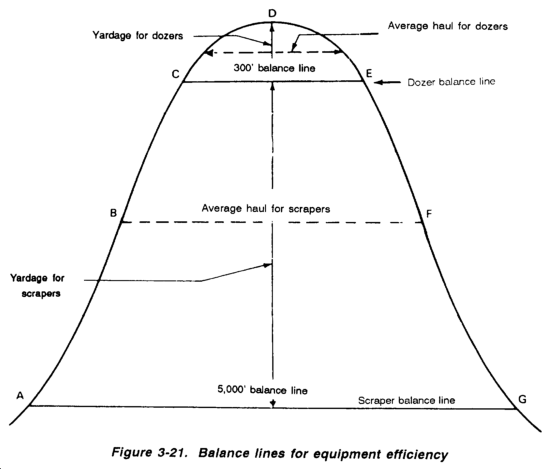
Because the lengths of balance lines on a mass diagram are equal to the maximum or minimum haul distances for the balanced earthmoving operation between their end points, they should be drawn to conform to the capabilities of the available equipment. Equipment planned accordingly will operate at haul distances that are within its best range of efficiency. Figure 3-21 illustrates a portion of a mass diagram on which two balance lines have been drawn: 300 feet to conform to dozer capabilities and 5,000 feet for the scraper.
The following job analysis can be made from the diagram in Figure 3-21:
Use dozers between stations C and E. The maximum haul distance is 300 feet; the average haul distance is the horizontal bisector shown. The amount that will be cut between stations C and D and filled between stations D and E is the length of the indicated vertical lines.
Use scrapers for cutting from stations A to C and filling from stations E to G. The minimum and maximum haul distances are 301 and 5,000 feet, respectively. The average haul distance is the horizontal line midway between the balance lines. The amount of earthwork is indicated by the vertical line.
To determine the average grade for either the scraper or dozer work area, use the following procedure:
1. Draw on the profile a horizontal line through the work area that roughly divides the area in half. This is a rough estimation. (See Figure 3-22.)
2. Extend a vertical line from the end points of the previously determined average haul line up through the project profile. These lines are referred to as the average haul vertical. (See Figure 3-23.)
3. Draw a final line connecting the intersecting points of the lines drawn in steps 1 and 2. This line represents the average grade.
4. Determine the average change in elevation (the vertical distance between the cut and fill).
5. Calculate the average grade as follows:
In the example shown in Figure 3-23, the average grade for the dozer would be--
Average Grade % =
Since this is an operation which moves earth downhill, the grade would be negative, or -8.87%. An uphill cut would have a positive grade.
Placement of Balance Lines to Minimize Work
Because the area between the apex of the mass diagram and the balance line is a measure of the work involved in the balancing operation, the size of these areas should be decreased whenever possible. However, the method used to minimize the area depends upon the shape of the mass diagram and the number of adjacent nodes that can be used.
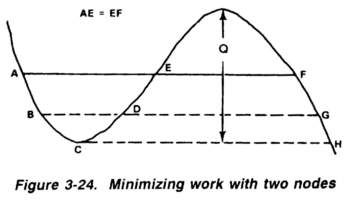
If two nodes are adjacent, work is minimized when two balance lines are drawn as one continuous line, with the balance lines equal in length. Each balance line must be within the maximum efficient haul distances for the equipment. The best placement of balance lines on the portion of a mass diagram shown in Figure 3-24 would be lines AE and EF, with AE = EF.
Only one balance line, CH, may be needed if it is within efficient haul distance specifications. The quantity involved would be Q yards and the work involved would be the area above CH.
If this one balance line was replaced by two balance lines, BD and DG, with BD less than DG. the quantity of earthwork balanced would remain the same. The work would be decreased by the size of the area between CH and DG and increased by the size of the area between BD and C. This would result in a savings because the increase is less than the decrease for the same amount of earthwork balanced. This decreasing process will continue by raising the lines to the point where one equals the other, or until AE = EF is reached.
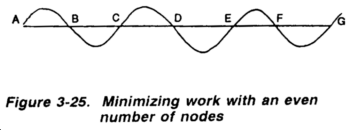
If there is an even number of adjacent nodes, as shown in Figure 3-25, work is reduced when the balance lines are one continuous line and AB + CD + EF = BC + DE + FG. The length of each balance line must be within equipment maximum haul capabilities as defined earlier.
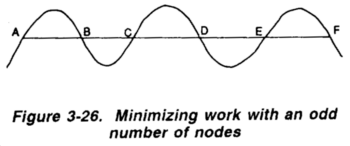
If there is an odd number of adjacent nodes, as shown in Figure 3-26, work is decreased when the balance lines are one continuous line and AB + CD + EF - (BC + DE) equals the limit of efficient haul, or approximately 1,000 feet. All balance lines must be within equipment limits.
Calculation of Earthwork not Within Balance Lines
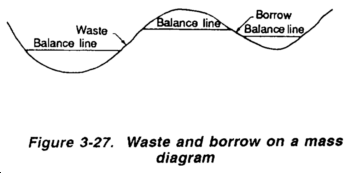
It is usually impossible to place balance lines so that the entire amount of earthwork on a project can be balanced. Some part of the mass line will be outside the balance lines. This material must be wasted or borrowed. If the portion not within balance lines is ascending (cutting), there is waste; if it is descending (filling), there is borrow. This is shown in Figure 3-27. Concentrate all necessary borrow and waste operations in one general area.
Format for Analysis
The simplest and most practical method of tabulating the results of a mass diagram is to write all quantities and distances on the diagram, as shown in Figure 3-28. It is also possible to extract information from the mass diagram and put it in a format that effectively controls the operation. One method is to prepare a mass diagram analysis sheet as shown in Figure 3-29.
LIMITATIONS OF THE MASS DIAGRAM
The mass diagram has many limitations that preclude its use in all earthmoving operations. At best, it is merely a guide indicating the general manner in which the operations should be controlled. Any attempt to get exact quantities and distances from it may be misleading. However, it is a good starting point.
The mass diagram is most effective when used to plan operations along an elongated project similar to a road, an airfield runway, or a taxiway. The haul distances are along the centerline or parallel to it. However, if the project becomes relatively wide compared to its length, movement of earth may be transverse as longitudinal, resulting in longer, transverse haul distances and invalidating the mass diagram analysis.
The mass diagram is used to analyze only the potentiality of balancing within one phase of a project. For instance, the mass diagram may indicate that the best balancing of a certain portion of a runway will require a haul distance of 2,200 feet along the site. However, it may be better to balance yardage with an adjacent taxiway in which the haul distance will be only 1,200 feet. The mass diagram can deal only with the runway or the taxiway, not with both simultaneously.
The mass diagram assumes that all material excavated in the cut sections is acceptable for use in the embankment sections. This is not necessarily true. However, all unacceptable quantities can be eliminated from the earthwork table.
The mass diagram is applicable to projects needing balanced earthwork. Balancing eliminates the double handling of quantities. If there is a short distance between an acceptable borrow pit and an embankment section, it may be more economical to use the borrow pit instead of a long balancing operation. This can be determined by a work or economy study.
|
NEWSLETTER
|
| Join the GlobalSecurity.org mailing list |
|
|
|